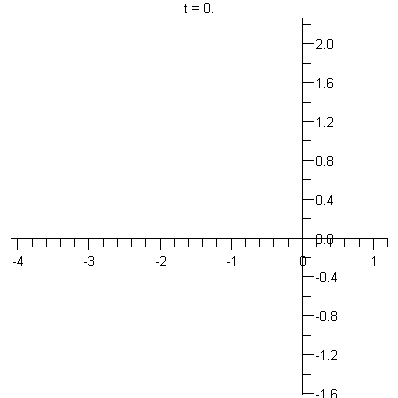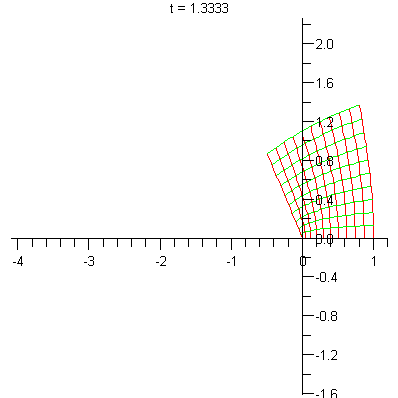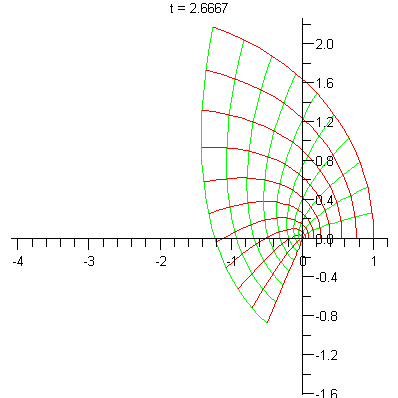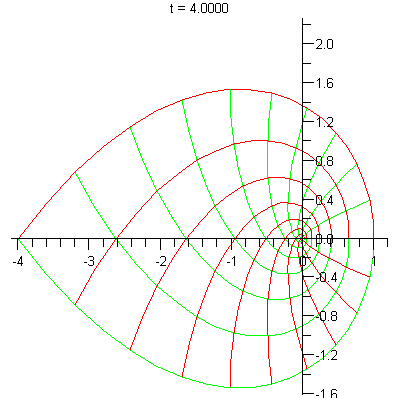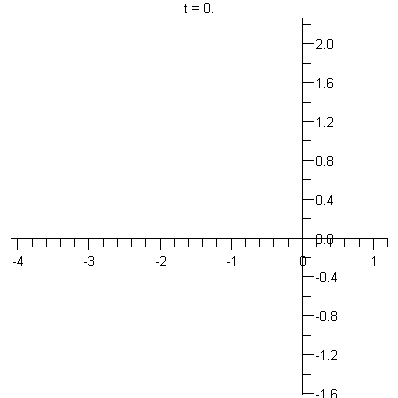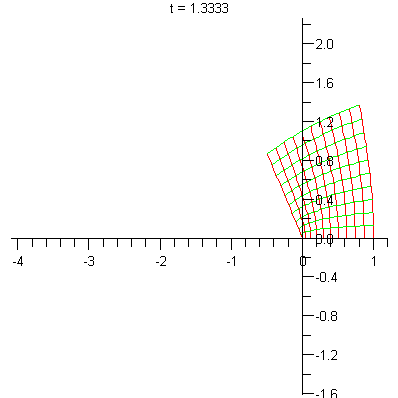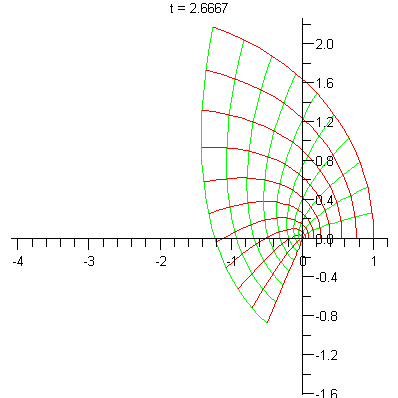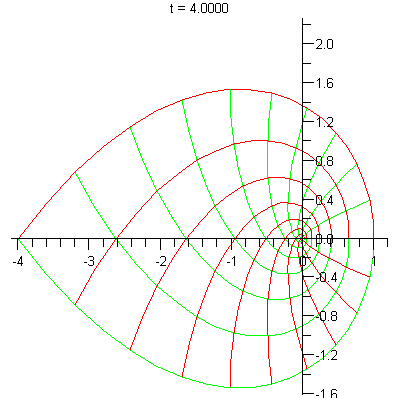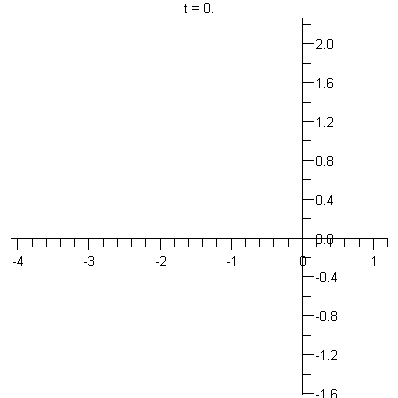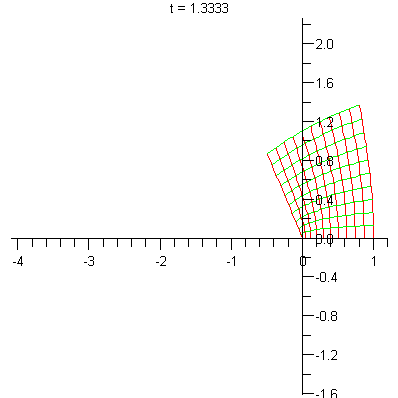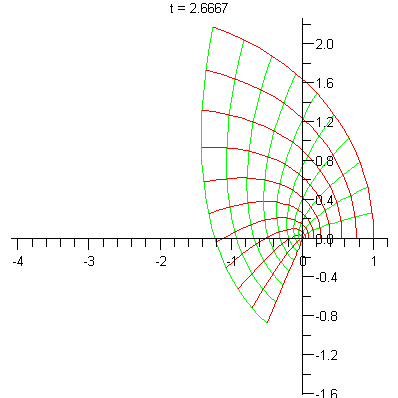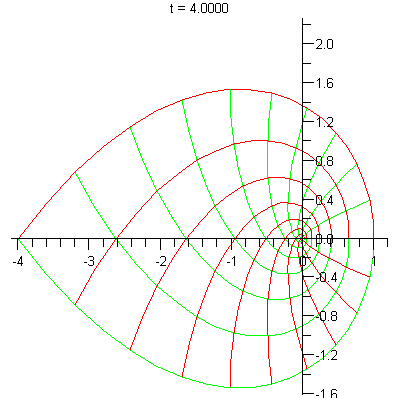Conformal Mapping
Home!
Conformal mappings
are what happens to complex numbers when you act certain functions
on them. (analytic functions, in particular) If you see what happens to certain
'input' curves, such as lines, or circles,
the result is often a very beautiful set of curves that preserve the angles where the
'input' curves cross.
What you see here is the image of a rectangular region in the complex plane,
acted upon by the analytic function zt, where t is just a real number
varying from 0 to 4 and z is any number in the domain region. I thought it'd be cute to animate it going from
0 to 4, as well as illustrative, so I worked up an animation in Maple.